How to Find the Phase Constant in Simple Harmonic Motion

F-kx where x is the displacement from an equilibrium position of. ω t ϕ A cos.

Phase Constant Youtube

If the total energy of the system is 20 joules find the force constant of the spring and the amplitude of the.

. By definition Simple harmonic motion in short SHM is a repetitive movement back and forth through an equilibrium or central position so that the maximum displacement on one side of this position is equal to the maximum displacement on the other side In other words in simple harmonic motion the object moves back and forth along a line. Simple Harmonic Motion Solutions 1. X is the displacement from the mean position A is the amplitude w is the angular frequency and φ is the phase constant.

At t 16s y 0 according to the graph. In this problem at time t 0 the position of the mass is x A. So the phase constant in a simple harmonic motion is measured in radians.

A t -ω 2 A cos ωt φ -ω 2 x. The difference of total phase angles of two particles executing simple harmonic motion with respect to the mean position is known as the phase difference. It is determined by the initial conditions of the motion.

The phase constant is an angle. Figure 156 shows a plot of the position of the block versus time. When changing values for displacement velocity or acceleration the calculator assumes the frequency stays constant to calculate the other two unknowns.

X t A cos ω t φ. A 175kg particle moves as function of time as follows. ω t ϕ So youd have to check both solutions.

The displacement of a particle performing simple harmonic motion is given by xAsinωtϕ where A is the amplitude ω is the frequency t is the time and ϕ is the phase constant. V d d t x A ω sin. Simple harmonic motion occurs when the restoring force is directly proportional to the displacement from equilibrium.

X A where A is the amplitude of the motion and T is the period of the oscillation. At t0 x0 and the particle is increasing its position. The equation of SHM is given by.

The pendulum executes simple harmonic motion and makes 100. The equation of the displacement is x 010cos10πt. This is the generalized equation for SHM where t is the time measured in seconds ω ω is the angular frequency with units of inverse seconds A is the amplitude measured in meters or centimeters and φ φ.

ω t ϕ ω where x is displacement A is the amplitude ω k m the angular frequency and ϕ the phase. Y 024mcos 71 s -1 t - 0095m. A 200g mass is attached to a spring and allowed to execute simple harmonic motion with a period of 025seconds.

The angle is measured in radians. The phase constant is also known as the epoch of the simple harmonic motion. Increase position is the same as saying possitive velocity.

Phase is a particular point in time on the cycle of a waveform measured as an angle in degrees. Yes I changed websites. Where δ is the phase constant of the simple harmonic motion which can be determined using the initial conditions.

Asked Oct 15 2019 in Physics by Shivam01 820k points oscillations. Finally the equation xt is given by. This tool calculates the variables of simple harmonic motion displacement amplitude velocity amplitude acceleration amplitude and frequency given any two of the four variables.

The period is the time for one oscillation. Hence the epoch is the initial phase of a particle performing the simple harmonic motion. Plugging this condition in xt.

If we consider an initial phase of a particle at t0 then the phase angle will be α. Hence option 3 is correct. X A sinωt ϕ where x is the distance from the mean position at any time t A is amplitude t is time ϕ is initial phase and ω is the angular frequency.

They start from mean position. Lets check the result. I know that after 2π the motion will repeat itself so it will not really matter but what is the conventional way to write the phase constant in the general equation of simple harmonic motion xA sin wt φ.

What is the phase of a wave. Two vibrating particles are said to be in the same phase the phase difference between them is an even multiple of π. Assuming an ideal spring the restoring force can be described as linear and obeying Hookes Law.

Two particles A and B execute simple harmonic motions of period T and 5T4. SPRING SIMPLE HARMONIC MOTION Purpose. To find the spring constant of a spring and determine the period of oscillation of a mass-spring oscillator in simple harmonic motion.

A What is the amplitude frequency angular frequency and period of this motion. The quantity φ is called the phase constant. X 4cos133tπ5 where distance is measured in metres and time in seconds.

Y A Sin ω t θ Where A is amplitude ω is the angular frequency t is time and θ is the initial phase anglephase constant. So you also need to work with the velocity formula which is the time derivative of the position. It is impossible for two particles each executing simple harmonic motion to remain in phase with each other if they have different.

This time translation invariance reappears here in the form of an arbitrary constant ϕ which acts as an arbitrary shift in time by ϕ. This αis called the phase constant of simple harmonic motion. π 2 and 3 π 2.

Figure 155 shows the motion of the block as it completes one and a half oscillations after release. ΔΦ nπ where n 0 1 2 3. Where F force and x the displacement from equilibrium.

When changing the frequency value the. X t A cos. 024mcos 71 s -1 16s - 0095m -001m.

If at t 0 the object has its maximum displacement in the positive x-direction then φ 0 if it has its maximum displacement in. The result is the same as the precision of the vertical scale and is therefore attributable to sighting error in.

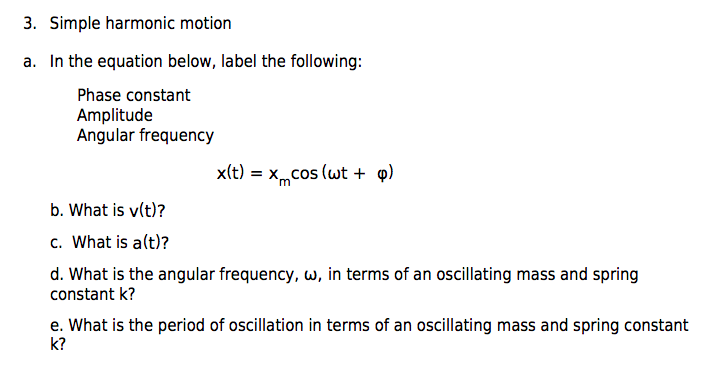
Solved 3 Simple Harmonic Motion A In The Equation Below Chegg Com

Physics Mechanics Ch 16 Simple Harmonic Motion 6 Of 19 Trig Equations W Phase Angle Youtube

2